Signal Multiresolution Analyzer
Decompose signals into time-aligned components
Description
The Signal Multiresolution Analyzer app is an interactive tool for visualizing multilevel wavelet- and data adaptive-based decompositions of real-valued 1-D signals and comparing results. The app supports single- and double-precision data. With the app, you can:
Access all the real-valued 1-D signals in your MATLAB® workspace.
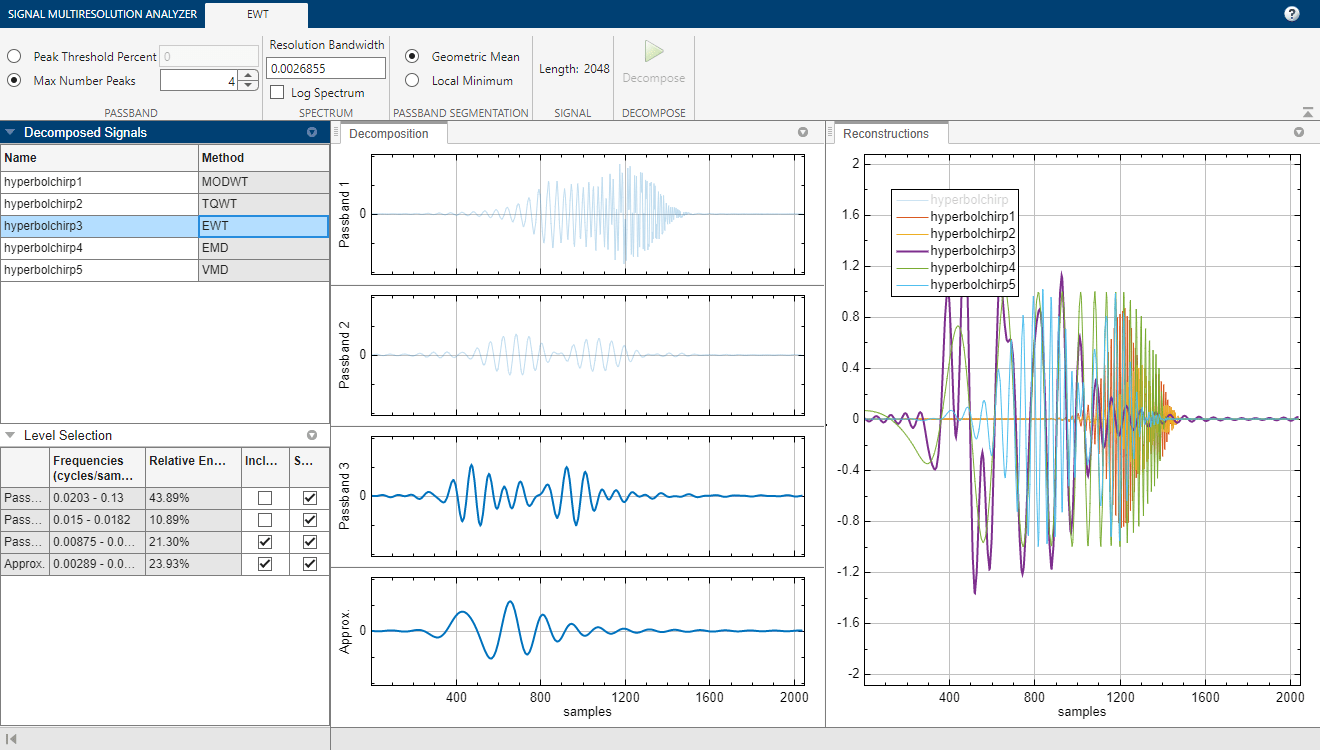
Generate decompositions using fixed-bandwidth and data-adaptive multiresolution analysis (MRA) methods:
Fixed-bandwidth: Maximal overlap discrete wavelet transform (MODWT) (default), and tunable Q-factor wavelet transform (TQWT)
Data-adaptive: Empirical mode decomposition (EMD), empirical wavelet transform (EWT), and variational mode decomposition (VMD)
Adjust default parameters, and visualize and compare multiple decompositions.
Choose decomposition levels to include in the signal reconstruction.
Obtain frequency ranges of the decomposition levels.
Determine the relative energy of the signal across levels.
Export reconstructed signals and decompositions to your workspace.
Recreate decompositions in your workspace by generating MATLAB scripts.
Open the Signal Multiresolution Analyzer App
MATLAB Toolstrip: On the Apps tab, under Signal Processing and Communications, click the app icon.
MATLAB command prompt: Enter
signalMultiresolutionAnalyzer.
Examples
Parameters
Programmatic Use
Tips
To decompose one channel of a multichannel signal, import the channel programmatically. For example, decompose the 10th channel of the multichannel Espiga3 EEG data set using these commands.
load Espiga3 signalMultiresolutionAnalyzer(Espiga3(:,10))To decompose different 1-D signals simultaneously, run multiple instances of Signal Multiresolution Analyzer.
For the MODWT and TQWT decomposition methods, the script generated by Signal Multiresolution Analyzer supports
gpuArray(Parallel Computing Toolbox) inputs.
Algorithms
References
[1] Percival, Donald B., and Andrew T. Walden. Wavelet Methods for Time Series Analysis. Cambridge Series in Statistical and Probabilistic Mathematics. Cambridge ; New York: Cambridge University Press, 2000.