Vehicle Steering Gain at Different Speeds
This example shows how to use the vehicle dynamics slowly increasing steering reference application to analyze the impact of the steering angle and speed on vehicle handling. Specifically, you can calculate the steering gain when you run the maneuver with different speed set points. Based on the constant speed, variable steer test defined in SAE J266, the slowly increasing steering maneuver helps characterize the lateral dynamics of the vehicle. In the test, the driver:
Accelerates until vehicle hits a target velocity.
Maintains a target velocity.
Linearly increases the steering wheel angle from 0 degrees to a maximum angle.
Maintains the steering wheel angle for a specified time.
Linearly decreases the steering wheel angle from maximum angle to 0 degrees.
For more information about the reference application, see Slowly Increasing Steering Maneuver.
helpersetupsis;

Run a Slowly Increasing Steering Maneuver
1. Open the Slowly Increasing Steer block. By default, the maneuver is set with these parameters:
Longitudinal speed setpoint — 50 mph
Handwheel rate — 13.5 deg
Maximum handwheel angle — 270 deg
2. In the Visualization subsystem, open the 3D Engine block. By default, the 3D Engine parameter is set to Disabled. Simulating models in the 3D visualization environment requires Simulink® 3D Animation™. For the 3D visualization engine platform requirements and hardware recommendations, see the Unreal Engine Simulation Environment Requirements and Limitations.
3. Run the maneuver with the default settings. As the simulation runs, view the vehicle information.
mdl = 'ISReferenceApplication';
sim(mdl);
### Searching for referenced models in model 'ISReferenceApplication'. ### Found 3 model reference targets to update. ### Starting serial model reference simulation build. ### Successfully updated the model reference simulation target for: Driveline ### Successfully updated the model reference simulation target for: PassVeh14DOF ### Successfully updated the model reference simulation target for: SiMappedEngineV Build Summary Model reference simulation targets: Model Build Reason Status Build Duration ================================================================================================================= Driveline Target (Driveline_msf.mexw64) did not exist. Code generated and compiled. 0h 0m 44.389s PassVeh14DOF Target (PassVeh14DOF_msf.mexw64) did not exist. Code generated and compiled. 0h 2m 12.619s SiMappedEngineV Target (SiMappedEngineV_msf.mexw64) did not exist. Code generated and compiled. 0h 0m 18.893s 3 of 3 models built (0 models already up to date) Build duration: 0h 3m 43.966s

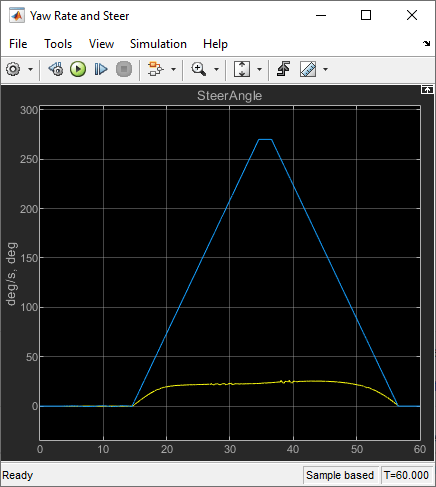
In the Vehicle Position window, view the vehicle longitudinal distance as a function or the lateral distance. The yellow line displays the yaw rate. The blue line shows the steering angle.
In the Visualization subsystem, open the Yaw Rate and Steer Scope block to display the yaw rate and steering angle versus time.
Sweep Speed Set Points
Run the slowly increasing steering angle reference application with three different speed set points.
1. In the slowly increasing steering reference application model ISReferenceApplication, open the Slowly Increasing Steer block. The Longitudinal speed set point, xdot_r block parameter sets the vehicle speed. By default, the speed is 50 mph.
2. Enable signal logging for the velocity, lane, and ISO signals. You can use the Simulink® editor or, alternatively, these MATLAB® commands. Save the model.
Enable signal logging for the Slowly Increasing Steer Ref signal outport.

mdl = 'ISReferenceApplication'; ph=get_param('ISReferenceApplication/Slowly Increasing Steer','PortHandles'); set_param(ph.Outport(1),'DataLogging','on');
Enable signal logging for the Passenger Vehicle block outport signal.
ph=get_param('ISReferenceApplication/Passenger Vehicle','PortHandles'); set_param(ph.Outport(1),'DataLogging','on');
In the Visualization subsystem, enable signal logging for the ISO block.
set_param([mdl '/Visualization/ISO 15037-1:2006'],'Measurement','Enable');
3. Set up a speed set point vector, xdot_r, that you want to investigate. For example, at the command line, type:
vmax = [45, 50, 55]; numExperiments = length(vmax);
4. Create an array of simulation inputs that set the Slowly Increasing Steer block parameter Longitudinal speed setpoint, xdot_r equal to xdot_r.
for idx = numExperiments:-1:1 in(idx) = Simulink.SimulationInput(mdl); in(idx) = in(idx).setBlockParameter([mdl '/Slowly Increasing Steer'], ... 'xdot_r', num2str(vmax(idx))); end
5. Save the model and run the simulations. If available, use parallel computing.
save_system(mdl) tic; simout = parsim(in,'ShowSimulationManager','on'); toc;
[26-Jun-2024 14:19:58] Checking for availability of parallel pool... [26-Jun-2024 14:19:59] Starting Simulink on parallel workers... [26-Jun-2024 14:20:03] Loading project on parallel workers... [26-Jun-2024 14:20:03] Configuring simulation cache folder on parallel workers... [26-Jun-2024 14:20:03] Loading model on parallel workers... [26-Jun-2024 14:20:32] Running simulations... [26-Jun-2024 14:21:37] Completed 1 of 3 simulation runs [26-Jun-2024 14:21:37] Received simulation output (size: 20.64 MB) for run 2 from parallel worker. [26-Jun-2024 14:22:08] Completed 2 of 3 simulation runs [26-Jun-2024 14:22:08] Received simulation output (size: 20.74 MB) for run 3 from parallel worker. [26-Jun-2024 14:23:38] Completed 3 of 3 simulation runs [26-Jun-2024 14:23:38] Received simulation output (size: 20.69 MB) for run 1 from parallel worker. [26-Jun-2024 14:23:38] Cleaning up parallel workers... Elapsed time is 233.647680 seconds.
6. After the simulations complete, close the Simulation Data Inspector windows.
Use Simulation Data Inspector to Analyze Results
Use the Simulation Data Inspector to examine the results. You can use the UI or, alternatively, command-line functions.
1. Open the Simulation Data Inspector. On the Simulink Toolstrip, on the Simulation tab, under Review Results, click Data Inspector.
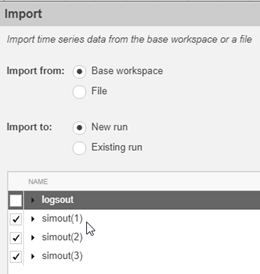
In the Simulation Data Inspector, select Import.

In the Import dialog box, clear
logsout. Selectsimout(1),simout(2), andsimout(3). Select Import.
Use the Simulation Data Inspector to examine the results.
2. Alternatively, use these MATLAB commands to plot the longitudinal velocity, steering wheel angle, lateral acceleration, longitudinal position, and lateral position.
for idx = 1:numExperiments % Create sdi run object simoutRun(idx)=Simulink.sdi.Run.create; simoutRun(idx).Name=['Velocity = ', num2str(vmax(idx))]; add(simoutRun(idx),'vars',simout(idx)); end sigcolor=[0 1 0;0 0 1;1 0 1]; for idx = 1:numExperiments % Extract the lateral acceleration, position, and steering msignal(idx)=getSignalsByName(simoutRun(idx), 'xdot_mph'); msignal(idx).LineColor =sigcolor((idx),:); ssignal(idx)=getSignalsByName(simoutRun(idx), 'SteerAngle'); ssignal(idx).LineColor =sigcolor((idx),:); asignal(idx)=getSignalsByName(simoutRun(idx), 'Lateral acceleration'); asignal(idx).LineColor =sigcolor((idx),:); xsignal(idx)=getSignalsByName(simoutRun(idx), 'Passenger Vehicle:1.Body.InertFrm.Cg.Disp.X'); xsignal(idx).LineColor =sigcolor((idx),:); ysignal(idx)=getSignalsByName(simoutRun(idx), 'Passenger Vehicle:1.Body.InertFrm.Cg.Disp.Y'); ysignal(idx).LineColor =sigcolor((idx),:); end Simulink.sdi.view Simulink.sdi.setSubPlotLayout(5,1); for idx = 1:numExperiments % Plot the lateral position, steering angle, and lateral acceleration plotOnSubPlot(msignal(idx),1,1,true); plotOnSubPlot(ssignal(idx),2,1,true); plotOnSubPlot(asignal(idx),3,1,true); plotOnSubPlot(xsignal(idx),4,1,true); plotOnSubPlot(ysignal(idx),5,1,true); end
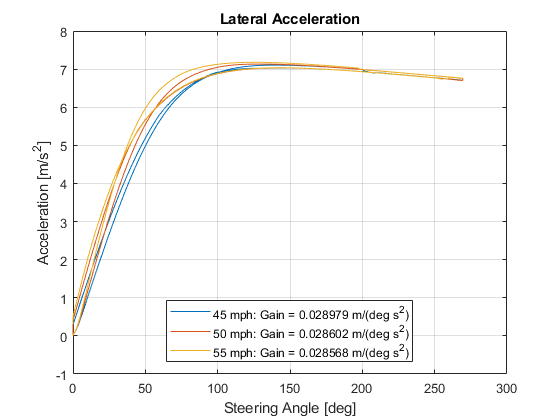
The results are similar to these plots, which indicate that the greatest lateral acceleration occurs when the vehicle velocity is 45 mph.

Further Analysis
To explore the results further, use these commands to extract the lateral acceleration, steering angle, and vehicle trajectory from the simout object.
1. Extract the lateral acceleration and steering angle. Plot the data. The results are similar to this plot.
figure for idx = 1:numExperiments % Extract Data log = get(simout(idx),'logsout'); sa=log.get('Steering-wheel angle').Values; ay=log.get('Lateral acceleration').Values; firstorderfit = polyfit(sa.Data,ay.Data,1); gain(idx)=firstorderfit(1); legend_labels{idx} = [num2str(vmax(idx)), ' mph: Gain = ', ... num2str(gain(idx)), ' m/(deg s^2)']; % Plot steering angle vs. lateral acceleration plot(sa.Data,ay.Data) hold on end % Add labels to the plots legend(legend_labels, 'Location', 'best'); title('Lateral Acceleration') xlabel('Steering Angle [deg]') ylabel('Acceleration [m/s^2]') grid on
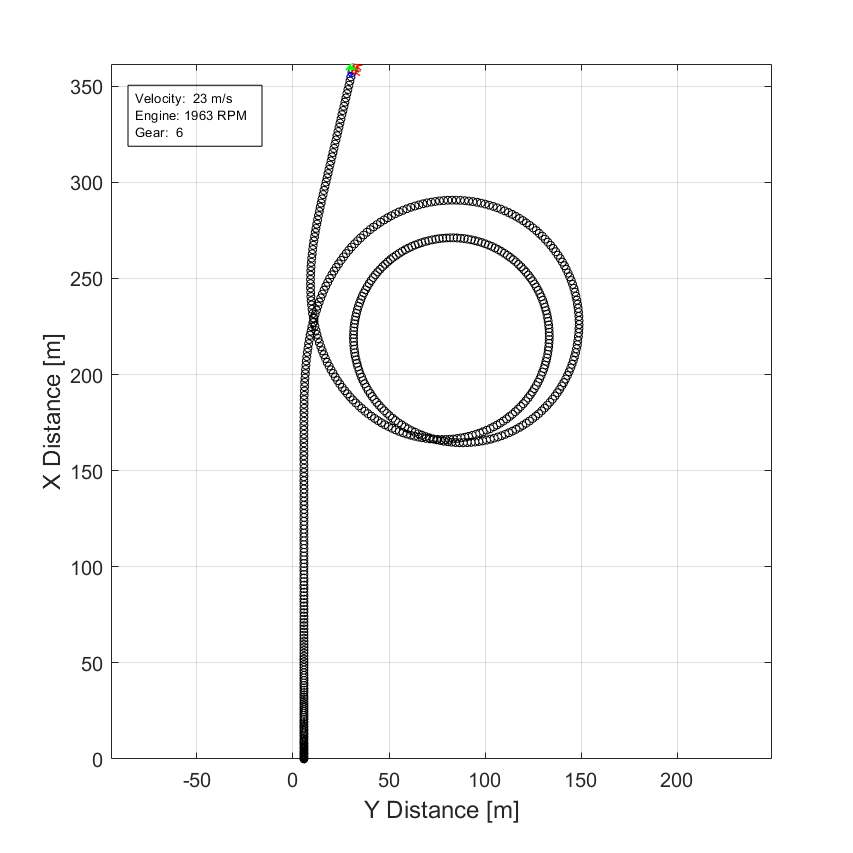
2. Extract the vehicle path. Plot the data. The results are similar to this plot.
figure for idx = 1:numExperiments % Extract Data log = get(simout(idx),'logsout'); xValues = getSignalsByName(simoutRun(idx), 'Passenger Vehicle:1.Body.InertFrm.Cg.Disp.X').Values; yValues = getSignalsByName(simoutRun(idx), 'Passenger Vehicle:1.Body.InertFrm.Cg.Disp.Y').Values; x = xValues.Data; y = yValues.Data; legend_labels{idx} = [num2str(vmax(idx)), ' mph']; % Plot vehicle location axis('equal') plot(y,x) hold on end % Add labels to the plots legend(legend_labels, 'Location', 'best'); title('Vehicle Path') xlabel('Y Position [m]') ylabel('X Position [m]') grid on
References
[1] SAE J266. Steady-State Directional Control Test Procedures For Passenger Cars and Light Trucks. Warrendale, PA: SAE International, 1996.
See Also
Simulink.SimulationInput | Simulink.SimulationOutput | polyfit
